- Poker Games
- Dice Poker Board Game
- Play Dice Poker Online With Friends – Free Skill Board Games!
- Online Poker Dice
- Dice Clubs (previously known as Dice Duel) is a classic competitive dice game with simple rules. It's a unique combination of luck, skill and strategy that you all know and love. Challenge your buddies or look for opponents online, start rolling dices and show them who's the master!
- Vintage Poker dice, taking dice in case, plastic gaming dice, vintage board game, vintage dice, board game gift idea. 5 out of 5 stars (402) $ 10.09. Favorite Add to 2pc Medium Size Galaxy Dice Charm - Punk charm -Resin Charm -Kawaii Charm -Solitaire -Poker.
On This Page
Introduction
Poker Dice is a game of chance available at Internet casinos using Wager Gaming Technology and 1x2 Gaming software. The game is based on rolling five poker dice and trying to form a paying poker hand.
Shake It Up - Dice Poker. 4,678 likes 15 talking about this. A fast, fun, head-to-head dice poker game!
Poker Games
Rules
- The player makes a bet.
- The game uses five poker dice. A poker die has on its six faces a 9, 10, jack, queen, king, and ace.
- The player is paid according to the poker value of the five dice, according to one of the following pay tables. All pays are on a 'for one' basis.
Wager Gaming Pay Table
| Hand | Pays |
|---|---|
| Five of a kind | 100 |
| High straight | 6 |
| Low straight | 5 |
| Four of a kind | 4 |
| Full house | 3 |
| Three of a kind | 2 |
| Two pair | 1 |
| Loser | 0 |
1x2 Gaming Pay Table
| Hand | Pays |
|---|---|
| Golden royal | 50 |
| Golden straight | 35 |
| Five of a kind | 20 |
| High straight | 6 |
| Low straight | 5 |
| Four of a kind | 4 |
| Full house | 3 |
| Three of a kind | 2 |
| Two pair | 1 |
| Pair of aces | 0.5 |
A Golden royal is a sequential and ascending straight to the ace. I guess there is a specific way they order the dice. A Golden straight is a sequential and ascending straight to the king. Dice in the correct order are shown in a golden color.
Wager Gaming Analysis
The following table shows the number of combinations, probability, and return from each outcome. The lower right cell shows a return of 97.99%.
Return Table
| Hand | Pays | Permutations | Probability | Return |
|---|---|---|---|---|
| Five of a kind | 100 | 6 | 0.000772 | 0.077160 |
| High straight | 6 | 120 | 0.015432 | 0.092593 |
| Low straight | 5 | 120 | 0.015432 | 0.077160 |
| Four of a kind | 4 | 150 | 0.019290 | 0.077160 |
| Full house | 3 | 300 | 0.038580 | 0.115741 |
| Three of a kind | 2 | 1,200 | 0.154321 | 0.308642 |
| Two pair | 1 | 1,800 | 0.231481 | 0.231481 |
| Loser | 0 | 4,080 | 0.524691 | 0.000000 |
| Total | 7,776 | 1.000000 | 0.979938 |
Dice Poker Board Game
1x2 Gaming Analysis
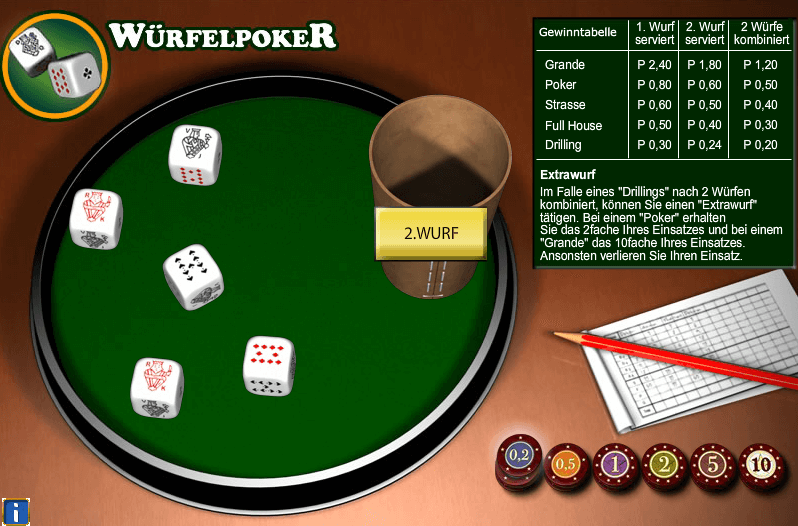
King billy casino. The following table shows the number of combinations, probability, and return from each outcome. The lower right cell shows a return of 96.63%.
Return Table
Play Dice Poker Online With Friends – Free Skill Board Games!
| Hand | Pays | Permutations | Probability | Return |
|---|---|---|---|---|
| Golden royal | 50 | 1 | 0.000129 | 0.006430 |
| Golden straight | 35 | 1 | 0.000129 | 0.004501 |
| Five of a kind | 20 | 6 | 0.000772 | 0.015432 |
| High straight | 6 | 119 | 0.015303 | 0.091821 |
| Low straight | 5 | 119 | 0.015303 | 0.076517 |
| Four of a kind | 4 | 150 | 0.019290 | 0.077160 |
| Full house | 3 | 300 | 0.038580 | 0.115741 |
| Three of a kind | 2 | 1,200 | 0.154321 | 0.308642 |
| Two pair | 1 | 1,800 | 0.231481 | 0.231481 |
| Pair of aces | 0.5 | 600 | 0.077160 | 0.038580 |
| All other | 0 | 3,480 | 0.447531 | 0.000000 |
| Total | 7,776 | 1.000000 | 0.966307 |
Math Lesson
I explain how the number of permutations of each hand in my page on Dazzling Dice, except the two pair, which doesn't pay in that game.
In a two pair, there are combin(6,2)=15 possible ways to choose two ranks out of five for the two pair. Then there are four ways left to choose the rank of the singleton. Then there are combin(5,2)=10 ways to choose the positions of the first pair. Then there are combin(3,2)=3 ways to choose the positions of the second pair. Thus, the total number of permutations for a two pair are 15×4×10×3 = 1,800.
External Links
- Poker Dice on the F1x2 platform — Specifications for the 1x2 Gaming version of Poker Dice. Without this, I'd have never known what a Golden Royal and Golden Straight were.
- What is a Golden Royal — Discussion about my frustration with determining what a Golden Royal is.

King billy casino. The following table shows the number of combinations, probability, and return from each outcome. The lower right cell shows a return of 96.63%.
Return Table
Play Dice Poker Online With Friends – Free Skill Board Games!
| Hand | Pays | Permutations | Probability | Return |
|---|---|---|---|---|
| Golden royal | 50 | 1 | 0.000129 | 0.006430 |
| Golden straight | 35 | 1 | 0.000129 | 0.004501 |
| Five of a kind | 20 | 6 | 0.000772 | 0.015432 |
| High straight | 6 | 119 | 0.015303 | 0.091821 |
| Low straight | 5 | 119 | 0.015303 | 0.076517 |
| Four of a kind | 4 | 150 | 0.019290 | 0.077160 |
| Full house | 3 | 300 | 0.038580 | 0.115741 |
| Three of a kind | 2 | 1,200 | 0.154321 | 0.308642 |
| Two pair | 1 | 1,800 | 0.231481 | 0.231481 |
| Pair of aces | 0.5 | 600 | 0.077160 | 0.038580 |
| All other | 0 | 3,480 | 0.447531 | 0.000000 |
| Total | 7,776 | 1.000000 | 0.966307 |
Math Lesson
I explain how the number of permutations of each hand in my page on Dazzling Dice, except the two pair, which doesn't pay in that game.
In a two pair, there are combin(6,2)=15 possible ways to choose two ranks out of five for the two pair. Then there are four ways left to choose the rank of the singleton. Then there are combin(5,2)=10 ways to choose the positions of the first pair. Then there are combin(3,2)=3 ways to choose the positions of the second pair. Thus, the total number of permutations for a two pair are 15×4×10×3 = 1,800.
External Links
- Poker Dice on the F1x2 platform — Specifications for the 1x2 Gaming version of Poker Dice. Without this, I'd have never known what a Golden Royal and Golden Straight were.
- What is a Golden Royal — Discussion about my frustration with determining what a Golden Royal is.
Online Poker Dice
Written by:Michael Shackleford

